`=>` Let us consider a function `f` given by `f(x) = x + 2,` `x ∈ (0, 1)`
`=>` we may note that the function even has neither a local maximum value nor a local minimum value in `(0,1).`
`=>` if we extend the domain of `f` to the closed interval `[0, 1],` then `f` still may not have a local maximum (minimum) values but it certainly does have maximum value `3 = f(1)` and minimum value `2 = f(0).`
`=>` The maximum value 3 of f at `x = 1` is called `"absolute maximum"` value (`"global maximum"` or greatest value) of `f` on the interval
`[0, 1].`
`=>` Similarly, the minimum value 2 of f at `x = 0` is called the absolute minimum value (global minimum or least value) of f on `[0, 1].`
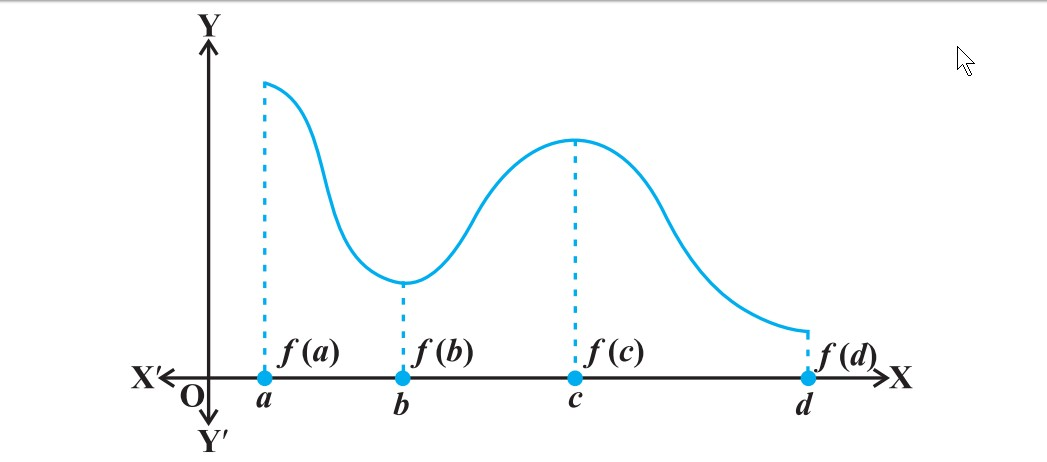
`=>` Consider the graph given in Fig, Observe that the function `f` has a local minima at `x = b` and local minimum value is `f(b).` The function also has a local maxima at `x = c` and local maximum value is `f(c).`
`=>` Also from the graph, it is evident that `f` has absolute maximum value `f(a)` and absolute minimum value `f(d).` and note that they different from local minimum and maximum value.
`\color{green} ✍️` Theorem : Let `f` be a continuous function on an interval `I = [a, b].` Then `f` has the absolute maximum value and `f` attains it at least once in `I.` Also, f has the absolute minimum value and attains it at least once in `I.`
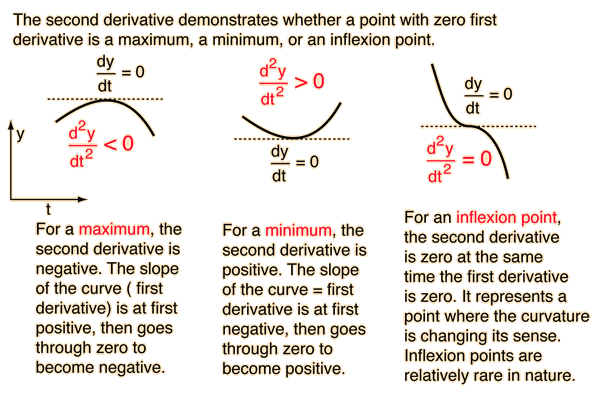
`\color{green} ✍️` Theorem : Let `f` be a differentiable function on a closed interval `I` and let `c` be any interior point of `I.` Then
(i) `color{blue}{f ′(c) = 0}` if `f` attains its absolute `"maximum"` value at c.
(ii) `color{blue}{f ′(c) = 0}` if `f` attains its absolute `"minimum"` value at c.
Working Rule :
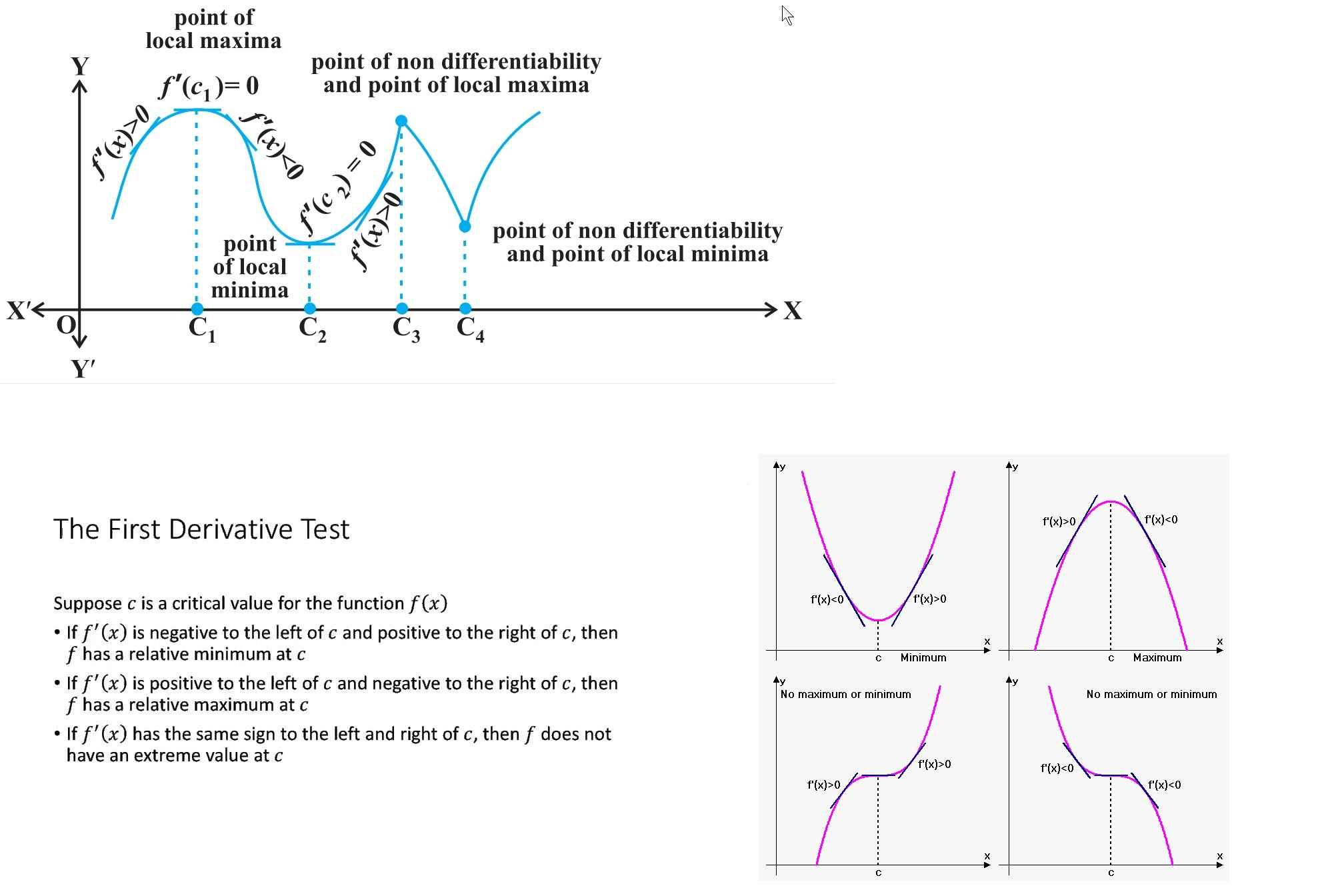
Step 1: Find all critical points of f in the interval, i.e., find points x where either `f' (x) = 0` or f is not differentiable.
Step 2: Take the end points of the interval.
Step 3: At all these points (listed in Step 1 and 2), calculate the values of f .
Step 4: Identify the maximum and minimum values of f out of the values calculated in Step 3. This maximum value will be the absolute maximum (greatest) value of `f` and the minimum value will be the absolute minimum (least) value of f .
`=>` Let us consider a function `f` given by `f(x) = x + 2,` `x ∈ (0, 1)`
`=>` we may note that the function even has neither a local maximum value nor a local minimum value in `(0,1).`
`=>` if we extend the domain of `f` to the closed interval `[0, 1],` then `f` still may not have a local maximum (minimum) values but it certainly does have maximum value `3 = f(1)` and minimum value `2 = f(0).`
`=>` The maximum value 3 of f at `x = 1` is called `"absolute maximum"` value (`"global maximum"` or greatest value) of `f` on the interval
`[0, 1].`
`=>` Similarly, the minimum value 2 of f at `x = 0` is called the absolute minimum value (global minimum or least value) of f on `[0, 1].`
`=>` Consider the graph given in Fig, Observe that the function `f` has a local minima at `x = b` and local minimum value is `f(b).` The function also has a local maxima at `x = c` and local maximum value is `f(c).`
`=>` Also from the graph, it is evident that `f` has absolute maximum value `f(a)` and absolute minimum value `f(d).` and note that they different from local minimum and maximum value.
`\color{green} ✍️` Theorem : Let `f` be a continuous function on an interval `I = [a, b].` Then `f` has the absolute maximum value and `f` attains it at least once in `I.` Also, f has the absolute minimum value and attains it at least once in `I.`
`\color{green} ✍️` Theorem : Let `f` be a differentiable function on a closed interval `I` and let `c` be any interior point of `I.` Then
(i) `color{blue}{f ′(c) = 0}` if `f` attains its absolute `"maximum"` value at c.
(ii) `color{blue}{f ′(c) = 0}` if `f` attains its absolute `"minimum"` value at c.
Working Rule :
Step 1: Find all critical points of f in the interval, i.e., find points x where either `f' (x) = 0` or f is not differentiable.
Step 2: Take the end points of the interval.
Step 3: At all these points (listed in Step 1 and 2), calculate the values of f .
Step 4: Identify the maximum and minimum values of f out of the values calculated in Step 3. This maximum value will be the absolute maximum (greatest) value of `f` and the minimum value will be the absolute minimum (least) value of f .